Technical Papers and Presentations

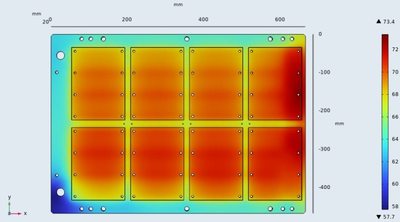
Pumped two-phase cooling for improving thermal management of IGBT in rail transportation has been investigated both experimentally and numerically. The experimental study on pumped two-phase cold plate of IGBT used in HXD1C locomotives was conducted at a mass flow rate of 0.1 kg/s to 0.29 kg/s and a heat flux range of 1.2 to 6.2 W/cm2, with R245fa as the working fluid. A 3-D numerical model for predicting cold plate base and IGBT case temperatures was established in the COMSOL Multiphysics® software. The Heat Transfer Module is used to model the solid 3D steady thermal conduction problem, while the EES (Engineering Equation Solver) software is invoked to define the convective heat flux boundary. By the combination of 3D and 1D simulation tools, i.e., COMSOL® and EES, the calculation efficency of temperature field has been dramatically increased. Given the heat losses of the IGBT module and the working conditions of two-phase flow, one can get the tempature profile within 1 minute, which is a surprising advantage for thermal monitoring. The simulation results have been compared with the counterpart of experimentation, the agreement is generally within an error band of 2 degC, which is satisfying in terms of engineering purposes. Furthermore, the numerical model has been compiled to be a standalone app according to the requirements of non-thermal experts. With the aid of this app, the eletric engineers and our customer can easily and instantly have a knowledge of the highest IGBT temperature, enabling precise control on the maximum power output to ensure safety. Such a modeling system and app distribution strategy profoundly changed the workflow of R&D in relevant business, such as PCB-based converter and water-cooled power module. Further work need to be conducted to meet the demands of transient temperature field prediction, since the heat loss of IGBT in rail transit applications is intrinsically time-dependent.