Technical Papers and Presentations

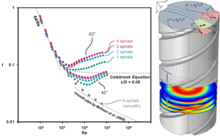
Partial differential equations (PDEs) in multiple dimensions may often be solved in a lower dimension if the problem domain contains a symmetry (cylindrical, spherical, translational, etcetera). For problems involving PDEs with helical symmetry we propose a method, with both low computational costs and low complexity, that reduces the domain to a 2D axial plane while retaining 3D Cartesian coordinates. A substitution rule for the partial derivative in axial direction is derived using the equivalence relation that relates the solution in the entire domain to the solution in the axial plane. As an example the substitution method is applied to the problem of turbulent flow in a helically symmetric corrugated tube.