Learning Center

How to Use 3D Geometric Primitives and Operations to Build 3D Geometry

---

There are several ways you can construct the geometry for a 3D model using COMSOL Multiphysics^®. You can draw and expand the 2D geometry into 3D, or you can directly build 3D geometric objects. This article focuses on the latter approach.

3D Object Primitives

The software contains several geometric operations, features, and functionality that enable you to create the geometry your model requires. This includes built-in primitive objects for many commonly used shapes, which you can use to quickly and easily generate geometric objects of different shapes. These include blocks, cylinders, cones, tori, helixes, and many more.

Some of the available shapes side by side, including a block, cone, sphere, pyramid, and helix, among others.

The 3D object primitives built into the software, which enable you to quickly generate many commonly used 3D shapes. See the settings for these shapes in the attached model file here. (Note that all primitives have been included in a single model file; this is done strictly for convenience. The use of multiple model components should only be done when it is motivated to do so.)

After adding the primitive object to your geometry sequence, the settings for it can be updated to have the dimensions and position, among other properties, that you require. Using these along with Boolean, transformation, and partition geometric operations enables you to build geometries, from simple to complex.

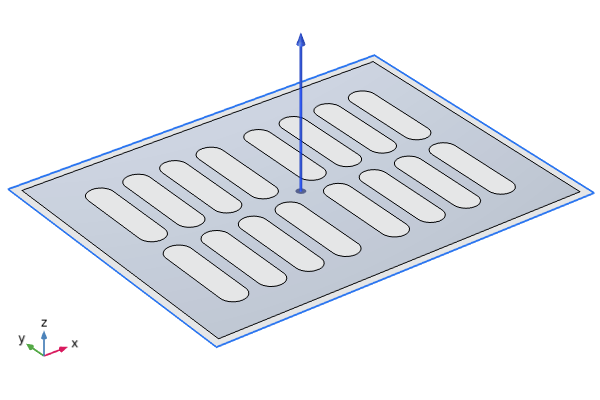
The COMSOL Multiphysics UI showing a geometry block selected in the Model Builder, the corresponding Settings window, and the micromixer model in the Graphics window.

The geometry for the micromixer tutorial model. In the geometry sequence, several blocks and hexahedrons are used to comprise the shape of the model.

Tutorial Video: Building Geometry Using 3D Object Primitives

Watch the video below and follow along using the software to learn step-by-step how to build a 3D model geometry, working entirely in the 3D space. We also give some tips for building geometries and discuss the many advantages of using parameters when building your model geometry.

Modeling Exercises

Put what you have learned here into practice with the follow-up modeling exercises shown here. Note that the directions are generalized to encourage self-guided problem solving. To check your work, you can reference the solution model files provided here or use the comparison tool to identify model differences.

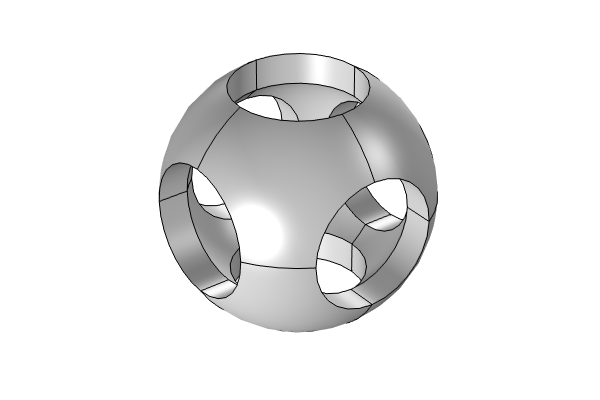
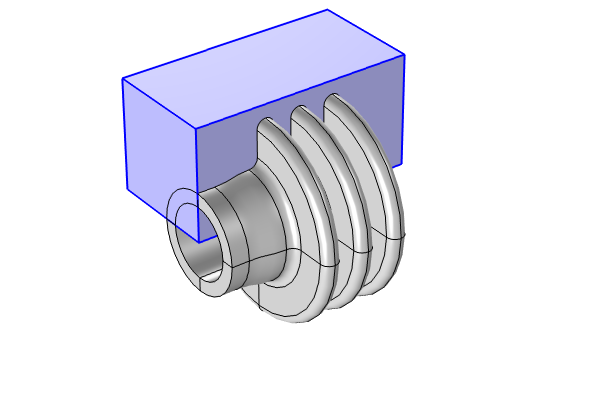
The 3D geometries for an elongated torus, hollow sphere with cutouts, and hollow cylinder with fins have been provided as downloadable MPHBIN files in this article. These MPHBIN files can be imported into COMSOL Multiphysics by selecting Import in the Home ribbon tab, Geometry ribbon tab, or the Geometry node. For each geometry, recreate the design from scratch in a 3D model component using 3D object primitives with geometry operations. You can measure the geometry to create a design with the same dimensions.

The geometry of an elongated torus (left), hollow sphere with cutouts (center), and hollow cylinder with fins (right).

There are a variety of ways the 3D geometry for each design can be obtained using COMSOL Multiphysics. We have outlined just one of these approaches for the practice models recommended here. Once you have tried building the geometry on your own, you can download the respective solution MPH-file to find additional guidance.

Further Learning

Throughout the tutorial models contained in the Application Libraries, 3D object primitives are used to build the model geometry. We encourage you to create the geometry for the tutorial models listed below to gain practice in building these shapes using the

Model	3D Object Primitives
Micromixer	• Hexahedron • Block
Electrical Signals in a Heart	• Sphere • Ellipsoid • Block • Cylinder
Loaded Spring	• Helix
Tuning Fork	• Cone • Sphere • Torus • Cylinder
Pyramidal Absorber	• Block • Pyramid
Rock Fracture Flow	• Parametric surface Note: find additional examples by searching @geom:ps in the Application Libraries